October 2010 ~ Easy-E

Sunday, October 31, 2010

Schaum's Outline of Electromagnetics

Here's your key to step-by-step mastery of electromagnetics— and better grades! This popular Schaum's Outline gives you completely worked problems, memorable examples, and easy-to-understand explanations of all relevant theory! Students love Schaum's Outlines! Each and every year, students purchase hundreds of thousands of the best study guides available anywhere. Students know that Schaum's delivers the goods in faster learning curves, better test scores, and higher grades!

Modified to conform to the current curriculum, Schaum's Outline of Electromagnetics complements these courses in scope and sequence to help you understand its basic concepts. The book offers extra practice on topics such as current density, capacitance, magnetic fields, inductance, electromagnetic waves, transmission lines, and antennas. Appropriate for the following course: Electromagnetics.

DOWNLOAD
DOWNLOAD
password: books_for_all

Tuesday, October 12, 2010

Green's Theorem

In mathematics, Green's theorem gives the relationship between a line integral around a simple closed curve C and a double integral over the plane region D bounded by C. It is the two-dimensional special case of the more general Stokes' theorem, and is named after British mathematician George Green.

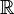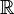Let C be a positively oriented, piecewise smooth, simple closed curve in the plane $\mathbb{R}$ ², and let D be the region bounded by C. If L and M are functions of (x, y) defined on an open region containing D and have continuous partial derivatives there, then

$\oint_{C} (L\, \mathrm{d}x + M\, \mathrm{d}y) = \iint_{D} \left(\frac{\partial M}{\partial x} - \frac{\partial L}{\partial y}\right)\, \mathrm{d}x\, \mathrm{d}y.$

For positive orientation, an arrow pointing in the counterclockwise direction may be drawn in the small circle in the integral symbol.

In physics, Green's theorem is mostly used to solve two-dimensional flow integrals, stating that the sum of fluid outflows at any point inside a volume is equal to the total outflow summed about an enclosing area. In plane geometry, and in particular, area surveying, Green's theorem can be used to determine the area and centroid of plane figures solely by integrating over the perimeter.

(From Wikipedia)